Abundant Recursive Mathematics Curricula Possibilities

• Author(s) / Creator(s)

  • Luo, Lixin

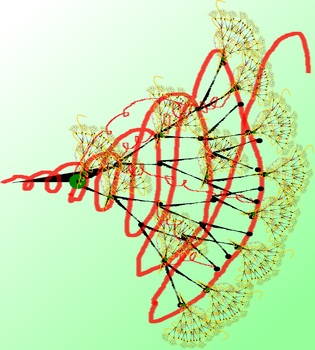
• A mathematics curriculum often seems be designed or delivered as linear: a sequence of predetermined, sometimes unrelated, topics with little chances for learners to revisit them from different perspectives. This suggests learning as accumulation with predictable outcomes. However, learning, observed through a complexfied worldview, is neither linear nor predictable. Learning is a self-organizing process through which a learner and her environment co-evolve, and a recursive elaboration through which a learner transforms her previous understanding. This view demands a recursive curriculum that changes along its formation. What might such curriculum be like in theory and practice is my research focus. This fractal-like image is a working visualization of recursive curricula. The nodes represent equivalent contents. Each branch represents certain mathematical/curricular event(s) that allow a content to change into or connect to another. The infinite spiral is made of loops that represent different development stages of an entry content (highlighted in green) and are ordered by the level of abstraction. The nodes on the same loop are different forms of the same content. Any node can be an entry point for many recursive curricula in the same or different dimension. This image represents abundant curriculum possibilities rather than a fixed one. // Program of study: PhD // Faculty/Department: Secondary Education // Place of creation: Edmonton, AB // Award: First Prize, Images of Research Competition 2019

• Date created

  2019-01-01

• Subjects / Keywords

  • Research image

• Type of Item

  Image

• DOI

  https://doi.org/10.7939/r3-7vek-hx75

• License

  Attribution 4.0 International